How Heavy Is the Air?
Figuring out the mass of Earth’s atmosphere via the back of an envelope

back-of-the-envelope calculation
A back-of-the-envelope calculation is an informal mathematical computation, often performed on a scrap of paper, such as an envelope. A back-of-the-envelope calculation uses estimated or rounded numbers to develop a ballpark figure quickly. The result should be more accurate than a guess, as it involves putting thought to paper, but it will be less accurate than a formal calculation performed using precise numbers and a spreadsheet or calculator.
Every now and then a question may bubble up, unbidden, into the front-row seats of your mind. A question to which you don’t know the answer. One that popped into my head a while back was “What’s the mass of the atmosphere?” (I don’t recall the context — or if there even was a context… as I said, unbidden.)
If you don’t know something, the web can offer you answers. But sometimes the answer itself is not the whole point — sometimes we run to work out, not to get from one place to another.
How much do we need to know to figure out the answer to this question? How much can we do off the top of our heads and in our heads? To work out the mass of the atmosphere we’re clearly going to need some numbers… but what numbers do we need? We don’t just need quantities: we need to know relationships between quantities.
May the force be with you
Let’s start with the relationship between mass and weight: something with a mass m has a weight mg. In everyday conversation we use the terms mass and weight interchangeably, and we use units of mass when we talk about weight (e.g., a kilogram is a unit of mass and, if you’re still using old money, a pound is defined as a unit of mass rather than of weight). Weight is a force and you may remember F = ma as the classical equation relating force to mass and acceleration. This is Newton’s second law of motion.
Even if you are at rest, you are still being accelerated towards the centre of the Earth. Fortunately, your fall is obstructed by the ground you’re standing on or the furniture you’re sitting on. Acceleration due to gravity is conventionally written as g, and so the force you experience as weight is mg. That mg applies to a person, to a building and to the whole atmosphere.
Although remembered by many as 9.81 ms⁻² (metres per second per second), g is not constant. g varies over the surface of the Earth depending on latitude (it’s greater at the poles than at the equator) and geology (unevenness of land as well as variation in density). Back-of-envelope calculations involves approximations for convenience so, interesting as all this is, we’ll just say g is 10 ms⁻².
We have seemingly replaced one question with another: what is the force of the atmosphere on the surface of the Earth? The difference between the two answers — the weight of the atmosphere versus the mass of the atmosphere — is a matter of moving a decimal point and changing units from newtons (N) to kilograms (kg). This doesn’t initially seem very useful until you realise that while you might not know the total force of the atmosphere over the whole surface area of the Earth, there’s a reasonable chance you might know the force per unit area, i.e., the pressure.
Under pressure
Pressure is something that is reported on weather apps and news sources. The only stumbling block to being able to apply what you can recall is the units. If you recall pressure as a manometric measurement, i.e., height of fluid in a manometer, you’ll need to know how to get from that to a measurement in SI units. Knowing that standard atmospheric pressure at sea level (1 atm) corresponds to 760 mm Hg (millimetres of mercury) is not immediately helpful when what we need here is a measurement in pascals (1 pascal = 1 Pa = 1 newton per square metre = 1 Nm⁻²).
If your recollection of atmospheric is in millibars, that’s much easier: 1 bar = 100 kPa (100 000 pascals). 1 atm is around 1013 mbar. To keep numbers — and recollection — simple, just remember that 1 atm ≈ 1 bar.
Going back to millimetres of mercury, if chemistry is your forte, you may know that mercury is a dense metal, ~13.5 times denser than water. Armed with that information you can work out pressure from a column of mercury 760 mm in height. On the other hand, if that 13.5 is not a piece of information you have to hand (I had to look it up), but you know the pressure in millibars, you can now determine the density of mercury, should that need ever arise when you’re pushing the back-of-the-envelope in future.
A more common issue issue is that some might recall atmospheric pressure in psi (pounds per square inch), which is dimensionally appropriate but incorrectly scaled for our needs. Keep in mind that Imperial units are all defined in terms of SI units. If you’re stuck using legacy units, it’s worth knowing the conversions to the base units those are actually defined in terms of, e.g., 1 foot is defined to be precisely 0.3048 metres (therefore, 1 in = 0.0254 m). The definition of pound requires more decimal places, but recalling the sides of food packaging (1 lb ≈ 454 g) or knowing the simple equivalence used in some languages that a pound is half a kilo (e.g., in German 1 Pfund = 500 grams) gets you the conversion you need.
Anyway, let’s head back to the bar. What does it mean to say that atmospheric pressure is around 100 000 pascals? That’s 100 000 newtons per square metre. Look at the floor around you and mentally mark out a square a metre on each side. Now imagine a column extending vertically upwards from that square into space: there’s around 10 000 kg (10 tonnes) of air in that column.
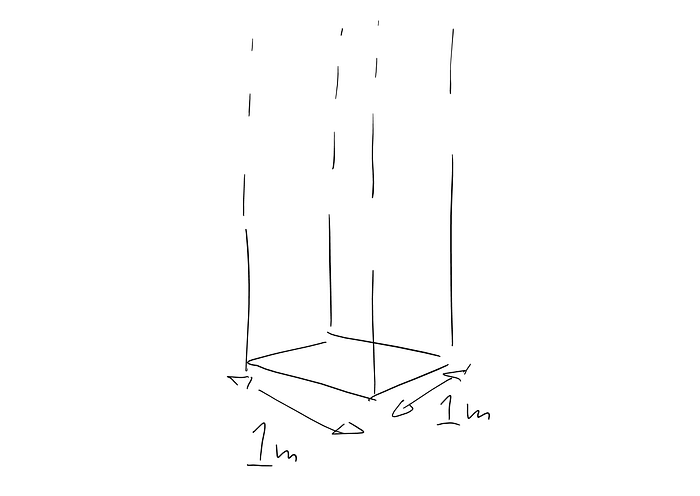
Area surveillance
Force relates to pressure as F = PA, where P is the pressure and A is the area over which the pressure applies. For an area of 1 m² we have F and, recalling that F = mg, dividing by g we have m. Multiply that by the surface area of the Earth and we have the mass of the atmosphere.
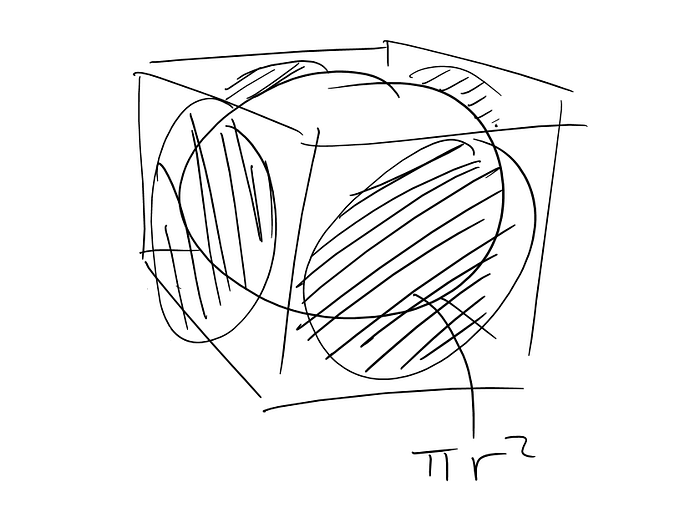
Although the Earth is a little flatter at the poles than at the equator, and there are ocean depths and mountain tops to consider in a full account, we don’t need to consider the Earth as anything but a sphere for this exercise (the radial deviation from a sphere is around 0.3%, but you can’t actually see the difference on a whole Earth photo even when you know it’s there). The surface area of a sphere is given by A = 4πr², where π plays its usual role as the ratio of a circle’s circumference to its diameter and r is the radius (half the diameter).
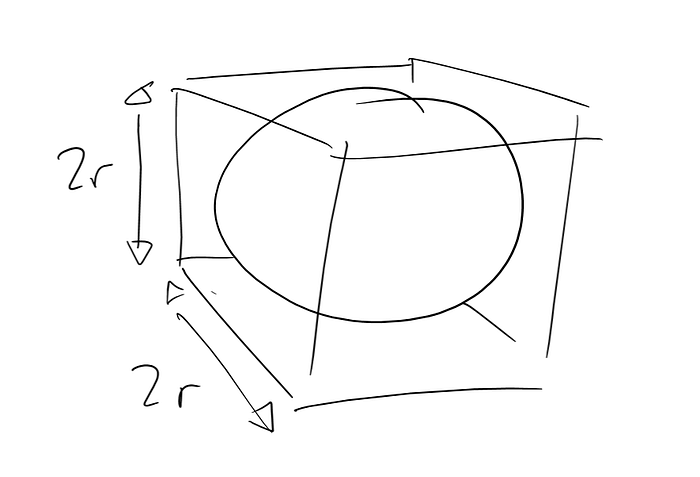
If that’s not a formula you recall, you could try treating the Earth as a cube. Each side of the cube equals the diameter (i.e., 2r) would give each face an area of 2r × 2r = 4r², so a total area of 6 × 4r² = 24r². This overestimates by almost a factor of two, but is still usable to get a result with the right order of magnitude.
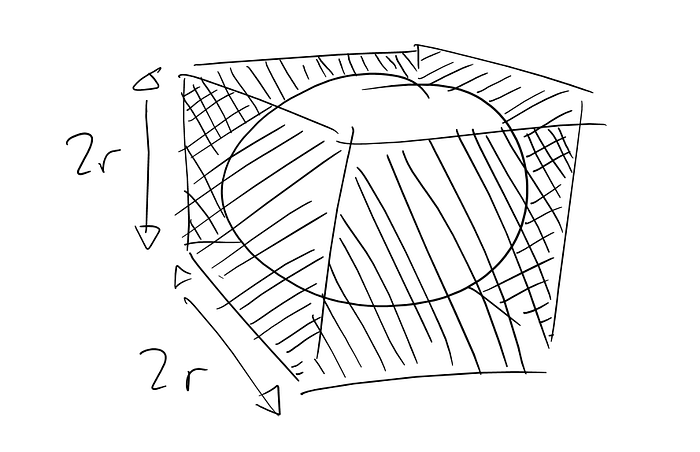
On the other hand, you might recognise that including all six sides puts it a long way over, and instead consider putting the Earth into a box without a top or bottom, i.e., four faces of a cube around the equator leaving the poles fully exposed. If you consider then folding the corners in towards the poles, you can see that you would be able to cover the surface of the Earth and have a little to spare. This would give an area of 4 × 4r² = 16r², which is still a little over, but is a better fit.
Alternatively, you may realise your wrapping skills don’t need a box but a cylinder to put the Earth in. The height of the cylinder is Earth’s diameter, 2r, and the distance around it is the diameter multiplied by π, i.e., 2πr. Therefore, the area would be 2r × 2πr = 4πr² — which is (1) exactly what we want and (2) not a coincidence.

Another way you could arrive at the same answer would be to consider looking at the projection of the Earth onto each of those four box faces: the area of the Earth’s circle on each face would be πr² and, therefore, the area of all four projections summed would be 4πr² — which is (1) again exactly what we want and (2) still not a coincidence.
This means all we need to do is figure out the radius of the Earth and we’re home and dry (or dry enough, given that around 70% of the Earth’s surface is covered by water). You might know this figure from as a matter of general knowledge or from another source. You may recall the Earth’s diameter as around 12 750 km (depending on how long you spent looking at tables of planetary facts as a child… but maybe that was just me), or more approximately as being around 12 500 km or 13 000 km. You may have come across the radius or diameter in a quote, for example, the opening chapter of Alice’s Adventures in Wonderland:
Down, down, down. Would the fall never come to an end! ‘I wonder how many miles I’ve fallen by this time?’ she said aloud. ‘I must be getting somewhere near the centre of the earth. Let me see: that would be four thousand miles down, I think — ’
Or you may know that the original definition of the metre was one ten millionth of the distance from the equator to the North pole over the Earth’s surface, i.e., whether longitude or latitude, north to south, east to west, the distance from 0° to 90° is around 10 000 km. Therefore, a great circle circumnavigation of the globe is, to within 0.2%, a 40 000 km trip.
If your knowledge is sourced in Imperial units, a convenient conversion coincidence is that miles to kilometres is roughly φ, the golden ratio, which is ~1.618 (the actual conversion is ~1.609). This means that, after skipping the first few values, successive pairs in the Fibonacci sequence (0, 1, 1, 2, 3, 5, 8, 13, 21…) offer a fairly good conversion (3 mi ≈ 5 km).
Let’s use the 40 000 km = 4 × 10⁷ m circumference figure, which is a convenient packaging of 2πr. This leaves us with 2r to figure out, which we can do by taking our 2πr and dividing it by π. As we are in the approximation business, we favour arithmetically friendly rational numbers over more precise and irrational ones. Using 3.2 (3⅕) as our value for π keeps things simple and sufficiently accurate, so 2r comes out as 1.25 × 10⁷ m (12 500 km). Multiplying our 2πr with our 2r gives us 5 × 10¹⁴ m² — five hundred trillion square metres — as the surface area of the Earth.
Mass effect
We calculated the mass per square metre earlier as 10 000 kg, which gives the total mass of the atmosphere as 5 × 10¹⁴ × 10⁴ kg = 5 × 10¹⁸ kg.
How does that compare with what we can find on the web? Pretty well:
- A 1966 paper in the Journal of Geophysical Research gives a value of around 5.136 × 10¹⁸ kg.
- The 2005 edition of the CRC Handbook of Chemistry and Physics also gives a value of around 5.136 × 10¹⁸ kg.
- A 2005 paper in the Journal of Climate gives the value as around 5.148 × 10¹⁸ kg.
Achieved by careful approximation, using what equates to the formula m = 4πr²P/g, our value of 5 × 10¹⁸ kg — five quintillion kilos — is within 3% of these more carefully and fully calculated values.
Had we abandoned simple arithmetic for more precise values (e.g., g as 9.80665 ms⁻², 1 atm as 101 325 Pa and π as 3.14159), while still retaining various approximations (such as a perfectly spherical Earth), this would have nudged the value up to around 5.26 × 10¹⁸ kg, slightly above the accepted values, reducing the proximity from just under 3% to a bit over 2%. This change is not a significant enough improvement to justify using more precision and heavier arithmetic in our approach.
We are often in awe of big questions. The common perception of science today is that it is large and expensive — particle physics, interplanetary probes, exoplanet hunting, etc. — and complex — quantum mechanics, climate modelling, gene editing — and this can deter us from seeing simplicity when it is present. In truth, many questions are often simpler than they appear, and the path to an acceptable answer may also be simpler than we believe.